Being a Liberal Means Never Having to Say You’re Forgiven
[UPDATE below.]
Now that Tom Woods and I have a hugely successful podcast analyzing Paul Krugman’s columns, I have hardly been paying attention to his blog posts. So while I was killing time today waiting for the Texas Tech parking lot to clear out after their heartbreaking buzzer-beater loss to Baylor, I got caught up on the Conscience of a Liberal. It reminded me why I’m not the man’s biggest fan.
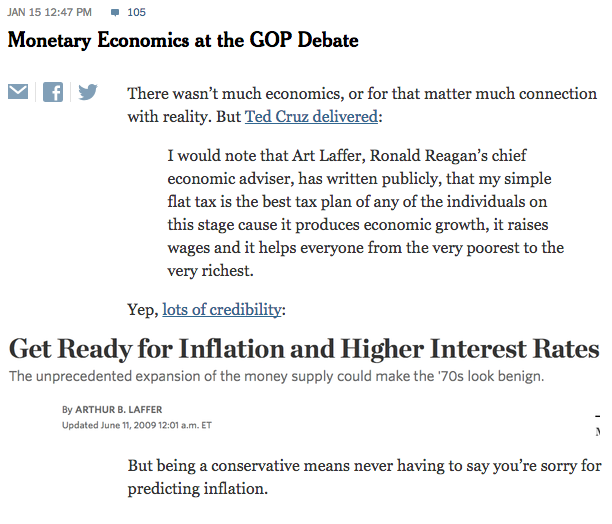
In order to make sense of his Jan. 15 post, I need to give you the screenshot:
So to be clear, Krugman is quoting Ted Cruz, then he’s posting a screenshot of a June 2009 Wall Street Journal column from Laffer predicting high inflation and interest rates, then Krugman comments, “But being a conservative means never having to say you’re sorry for predicting inflation.”
Now you know what’s funny about that? Two things. First, Ted Cruz was invoking Arthur Laffer’s authority on supply side tax cuts. That has absolutely nothing to do with monetary policy, and there is no one in human history more qualified to tell Republican voters which candidate’s plan is best from a supply-side perspective.
Second, Arthur Laffer did publicly acknowledge that his inflation predictions were way off, such that he would rethink his model. In other words, he did exactly what Krugman has been demanding such people do. And you can’t say, “Well, maybe Krugman missed that,” because Krugman publicly praised Laffer for doing so–in a blog post titled, “In Praise of Art Laffer,” and it was only two years ago. So you’d think Krugman might remember that one.
It’s because of stuff like this that it’s clear to me Krugman is completely insincere in his moral posturing and pleas for intellectual honesty. Yes, if writers (like me) made erroneous predictions about price inflation or anything else, we should be honest with our readers about what we think happened and how we’re trying to avoid such mistakes going forward. But the point certainly isn’t to curry favor with guys like Krugman. The only way to get on his good side is to switch to his policy prescriptions, which is why Kocherlakota is a good guy now.
UPDATE: After I posted the above, I saw at EconLog that David R. Henderson had similar remarks, though he pointed out Krugman’s (with Larry Summers) own warnings of an inflation time bomb (the actual phrase they used) in the early 1980s.







David Henderson has also pointed to Krugman’s early 80s paper forecasting high inflation in the years to come.
This comment was before the update (I think).
Right. (By the same token, I hadn’t see your comment when I made the update.)
CPI tracks the price of stuff POOR people purchase. POOR people do not have more money, therefore prices of stuff POOR people purchase, cannot increase. NO NEW SPENDING MONEY means NO price increase.
Let us instead track the price of vacation homes, Ferraris, etc.
Please think about what I am trying to share with you.
The main think I love about Keynes was his quote, ‘When the facts change I change my mind.’
The only people who do not do so are motivated purely out of belief rather than fact. We must ALL guard against dogma and instead seek to understand if something is correct or not. I can see evidence that portions of Keynes thoughts help give understanding to economics. Even Krugman has added a bit of ‘truth’ to the equation in describing liquidity and a liquidity trap, which has a great deal of merit to my way of thinking.
Also I would suggest that inflation did occur, what it did was mask the deflation that should have been occurring. You could say that it was ‘rampant’ inflation because we should have experienced ‘rampant’ deflation.
Just my take as to why the calls in 08 – 10 were wrong. But I would need to go more in depth to prove it.
Credit money was expanded therefore there was inflation. Credit money inflation and the ensuing price increase did not necessarily manifest itself at the retail level or within CPI. It did manifest itself in other areas.
To say there is no inflation is to say the money supply has not expanded.
Yet the money supply has expanded by all measures.
https://research.stlouisfed.org/fred2/series/WSAVNS
https://research.stlouisfed.org/fred2/series/BOGMBASE