Federal Government Changes Own Recommended Discount Rate When It Comes to Climate Change
[UPDATE below.]
As I explained when the May update came out, the Obama Administration’s “working group” on the “social cost of carbon” reports figures that vary crucially depending on the discount rate we choose. According to the updated document, the negative externality associated with one ton of carbon dioxide emissions in the year 2010 was:
==> $11/ton at a 5% discount rate,
==> $33/ton at a 3% discount rate,
==> $52/ton at a 2.5% discount rate.
You can see how much the discount rate matters on this issue. (The reason is that the computer-simulated damages don’t really start accruing until several decades in the future.)
Now this raises the interesting question of, “Which discount rate should the government use?” Oh if only there were some guidance!
Fortunately, the Office of Management and Budget (OMB) provided the following guidance in its 2003 update to Circular A-94:
2. Real Discount Rates of 3 Percent and 7 Percent
…
As a default position, OMB Circular A-94 states that a real discount rate of 7 percent
should be used as a base-case for regulatory analysis. The 7 percent rate is an estimate of the
average before-tax rate of return to private capital in the U.S. economy. It is a broad measure
that reflects the returns to real estate and small business capital as well as corporate capital.
…
The effects of regulation do not always fall exclusively or primarily on the allocation of
capital. When regulation primarily and directly affects private consumption (e.g., through higher
consumer prices for goods and services), a lower discount rate is appropriate. The alternative
most often used is sometimes called the social rate of time preference. This simply means the
rate at which “society” discounts future consumption flows to their present value. If we take the
rate that the average saver uses to discount future consumption as our measure of the social rate
of time preference, then the real rate of return on long-term government debt may provide a fair
approximation. Over the last thirty years, this rate has averaged around 3 percent in real terms on
a pre-tax basis. For example, the yield on 10-year Treasury notes has averaged 8.1 percent since
1973 while the average annual rate of change in the CPI over this period has been 5.0 percent,
implying a real 10-year rate of 3.1 percent.For regulatory analysis, you should provide estimates of net benefits using both 3 percent
and 7 percent. An example of this approach is EPA’s analysis of its 1998 rule setting both
effluent limits for wastewater discharges and air toxic emission limits for pulp and paper mills.
In this analysis, EPA developed its present-value estimates using real discount rates of 3 and 7
percent applied to benefit and cost streams that extended forward for 30 years. You should
present a similar analysis in your own work.
I have been in close contact with various people who go through all these releases with a fine-tooth comb, and none of us has yet found an estimate of what the SCC would be, at a 7 percent discount rate, even though that is part of the default regulatory requirement.
Also, if you want to argue that we should use Treasury yields, OK–tell me what the yield is on a (hypothetical) 75-year Treasury bond, because that’s (at least) the time horizon involved in climate change regulations and taxes. If we interpolate from the existing Treasury yield curve, it’s not obvious what the yield should be, but one could argue it’s more than 3 percent–especially if we think we’ll get out of the second Great Depression by 2050.
UPDATE: The last time I posted on this, somebody in the comments said the SCC couldn’t possibly be negative, regardless of the discount rate. Well, look at this figure from page 14 of the May 2013 update to the White House Working Group’s estimate:
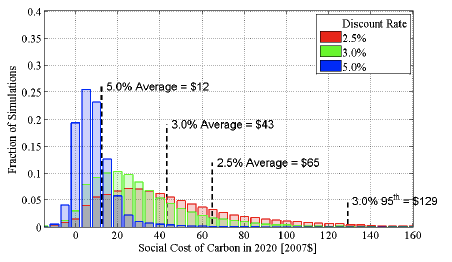
At just a 5% discount rate, the “average” SCC is reported as $12/ton. (And that’s for a ton of emissions in 2020, when the SCC will be higher than it is right now.) But if you look at those blue bars, you’ll see that this is because around 20% or so of the computer runs (at this discount rate) yield a SCC that is nonpositive, but which is counterbalanced by the 80% or so runs that have a positive SCC.
As you can see from the distribution of the green and red bars, throwing in a 7% discount rate would result in even more probability weight falling on the $0 or negative scenarios. Would the overall result be negative? I don’t know for sure without seeing the actual annual numbers, but the OMB default rule means that the Working Group should have reported that figure.







I did a quick plot of Avg SSC vs discount rate. Only 3 points, so it doesn’t mean much, but the data suggests there to are a lot of iterations involved with generating those 3 data points.
Anyway, it looks like the average would stay above zero, which isn’t surprising when you look at the distributions. The model seems to not have much variation to lower side. Look at how short the tail is on the low end of SCC for the 2.5% rate.
Also notice how far the averages are from the peaks of the curves. That may be fine, but it would make me want to look for any potential bias in the model.
Ken P. sorry but I don’t get what you are saying here. It looks like as you increase the discount rate, the frequency distributions get squished to the left. Going to 7% could quite easily lead to a negative average SCC, I would think.
What I did was plot the points (2.5,65), (3,43), (5,12) and looked at where various “out of the box” Excel trendlines pass through the point (7,y) .
Logarithmic and linear trendlines have negative SCC (around -$14 and -$29 respectively). Power and exponential trendlines stay slightly positive (around $5 and $3 respectively) and and appear to fit better.
Of course, there are many more possibilities for the curve shape. One more data point would give us a better idea, but like you say the guidleines ask for calculating a 7 percent discount rate. It makes me wonder whether someone calculated it and didn’t like the result.
Bob, I didn’t say it couldn’t possibly be negative. I merely pointed out that a quick and dirty plot of the three points suggested 7% might fall in the $3-5 range.
Here’s a problem with their data.
The “average” for blue is way different than the median. For the blue bars, 75% of the points are lower than the average. The cause is that long tail that was bothering me. Rare high value runs are causing a big effect on the average value.
There may be a good argument for using the average instead of the mean, but it’s generally problematic when your least likely results contribute significantly to your results.
I would expect that to be true of pretty much any interlocking system where you have a small but real probability of large and catastrophic failure when a number of fail safe protections all coincidently don’t work that day (like the Quebec train derailment).
In contrast then chance of a random chain of coincidence having an exceptionally good outcome, is practically zero.
Having pointed that out, I personally don’t believe the Earth’s climate is such an interlocking system, but possibly if you consider all agriculture and the way humans relate to the climate it might be. I also would regard the normal random weather fluctuations that we somehow deal with every day as much larger and more significant than the half a degree per century of warming that we maybe can measure (but many of the longest running thermometer series don’t measure even this).
yeah,. that makes sense. Good analogy with the train derailment. Something left out in this is analysis of the impact of a tax. I would expect a long tail on that due to potential disruptions of innovation.
Bob, I didn’t say it couldn’t possibly be negative.
I was talking about someone from a previous post, who was indeed saying it couldn’t be negative.
Gotcha. Thanks for the clarification.
Whilst ILGS remain available as an investment, it is recorded that the three year average yield has declined from 2.46% in 2001 to 0.2% in mid-2011, both before tax. It is further noted that, whilst periodical payments are available to claimants, the majority of awards still are in the form of a lump sum payment at the conclusion of the claim. It therefore is described as a risk that the current discount rate, given the decline in ILGS yields, is too high to give effect to the principle of 100% compensation and so it is appropriate the rate should be reviewed.
Bob, the 7 percent is the discount rate for return on investments. SCC pertain to the reduction in future welfare by an increase of emissions now with regard to consumption (and has a crucial intergenerational aspect). The discount rate on consumption is 3 percent, as even the paragraph you quote says. This is discussed in some detail later. Even the most one-sided reading of this would only say that a 7 percent discount rate should be included, but that they should go down to much lower (I didn’t see you complain that they didn’t use a 1 percent discount rate!). You will find this here by some relevant names insightful (or not, if you chose to further remain ignorant on this topic):
https://www.sussex.ac.uk/webteam/gateway/file.php?name=wps-56-2013.pdf&site=24
As I said in my original post: seven percent in this context seems high. If you have any past estimate were this has actually been used – in bright, blinking letters: IN THIS CONTEXT – please inform me.
The distribution you show has nothing – nothing – to do with a positive SCC estimate becoming negative by disounting. It simply reflects the fact that in some RUNS the SCC are actually negative – as the text clearly states! The result of a run is not an estimate: an estimate is the mean, or median etc that you get out of a series of runs. As you can see, they are ALL positive. What you show here is a simple histogramm showing how many runs yielded certain SCC values – as the text explains and the histogra
Please note that you are failing on a highschool level here: if you discount a value y > 0 at time t, your discounted present value with a discount rate r > 0 is simply y/((1+r)^t). Every expression here is positive, this cannot become negative, for purely mathematical reasons. As the discount rate is always positive (eventual exception: a negative growth rate), the value at time t itself must be negative if the present value is to be negative. I plotted a generic ‘value’ of unity with discount rates of 3, 30 and 300 percent for you, so that you can look at what happens here (it does not get negative, for example):
http://postimg.org/image/vtn1fcrwv/
Also note that even if I had made a mistake (which happens more often than not), your original reasoning would not have been sound: these negative values would have been calulated by mathematical magix, because the SCC are still not discounted sunk costs, as you apparently believe. Please update your knowledge of these issues – i.e. highschool maths and basic climate change economics – if you want to teach anybody anything about it.
I am also amused by your seemengly dismissive reference to models. Uncertainty leads to higher SCC. Another model failure is also rooted in uncertainty – just say “fat right tails”. As far as I remember you do not like the Weitzman assessment of these issues. So better go along
Do something about your knowledge gaps. Your mistakes and apparent failure to grasp these topics even on the most basic level do not go away by taking cheap shots at me (or “one commenter) or anybody pointing out were you have been mistaken. You have a PhD and every attempt to inform the public therefore comes with a certain responsibility to actually know what you are talking about and to get things right as good as you can. And pertaining to this topic, you are clearly clueless. Silly fake gotchas that just further expose your lack of knowledge don’t score you any points. Do your friggin homework.
Martin wrote:
Please note that you are failing on a highschool level here:
Martin, you’re the one looking at that chart and telling me that using a high enough discount rate couldn’t possibly push the average SCC negative. Yes, the average is an average of runs, and more runs turn out negative, the higher the discount rate. That’s what the chart shows.
No, you apparently believe that there is a series of runs (at which discount rate?) that are discounted ex post. This is not the case: as the text explains, for each run 150 000 runs have been, well, run. You can’t simply compare these runs and conclude that your preferred factor pushed those estimates from positive to negative – or only, if you moved goalposts. You are just interpreting you prior into the graph. The discount rate didn’t push those values negative (if so, you should be able to explain how): these values have been negative from the get-go, discoutning has only made them less negative. You can’t teach stuff based on personal impressions staring at a graph.
If this is still based on the notion that SCC are calculated by discounting sunk benefits and costs, read this here to see how SCC are actually calculated. :
http://www.sciencedirect.com/science/article/pii/S0095069610000422
The only idea I have how the discount rate could push SCC negative, is via the calibration of the damage function. If damage costs accrue more slowly, its slope might be lower, with the implication that more model runs fall in the negative range, statistically speaking (please take into account that this holds under the assumption that there is a real damage, i.e. that the damage fucntion is convex and sloping upwards). However, this has still nothing to do with the discount rate making a negative out of a positive. And however again, I simply do not know any of the IAMs well enough to make this more than a simple speculation (and at least you should be above speculation level, if you want to inform the public). And however the third, even under this assumption, this would only give you a higher fraction of runs in the negative and, with a very high discount rate, quasi-zero costs, while mean, median etc. would still be positive as long as you do not assume that a high discount rate has an influence on real climate change related impacts (or, put differently, made the derivative of the damage functions somehow change sign).
Martin, you agree with me that as we increase the discount rate, a higher proportion of runs turns out negative, right? And then, since “the” SCC for a given discount rate is the average across all the runs, “the” SCC gets pushed lower, at higher discount rates?
So why are you saying that no matter how high the discount rate, this process can’t lead to enough runs becoming negative, such that the overall average turns negative?
First, no: I do not agree. This your impression of one graph. If this is a systematic effect or if there just happened to be more runs with negative SCC with the 5 percent discount rate, is not clear. I don’t have the slightest idea as to how probable this is. Tell me, if you do, without handwaving. (I actually think it’s systematic, as there have been quite a few runs, but that’s besides the point). If you want to know that, you really have to check with FUND, DICE and PAGE (the latter might be a problem, Chris Hope only passes it over for research related reasons), or at least with one with them. As it stands, you just believe something and jump at a graph that somehow doesn’t contradict that believe, though it is also compatible with other interpretations (see below) and therefore does not confirm it, either. And this is independent of the question if the effect is systematic or not. This is no way to go for somebody who claims to have some insight, especially as you act under the odd idea that this has somehow escaped all economist in the field. As far as it concerns the two of us, these models are entirely blackbox models. None of us should make conclusions based on impressions that are not tested and we cannot test. But there are limits to what is plausible or and bounds to what is possible, regardless of model specifications.
That is, there are several possibilites to interpret your claim now that you ahve modified it, two are impossible or implausible, and the other is – as far as it concerns our expertise – untestable gobbledygook:
a) First, you can assume that SCC is the (negative) present value of global warming summed up from now to some point in the future. This is wrong, without any doubt: it is actually the net present value of the imptact on welfare of a further increase in emissions. That is, neither is it (directly) dependent on temperature (for that you have to take the transient climate response into account), nor is it a total cost (as even the per tonne unity tells you). So, any argument based on the notion that SCC can become negative by putting more weight on earlier net benefits, is nonsensical. Period. I remind you that this was your original claim, and I can quote it.
b) The idea that a future damage can be turned into a present gain by discounting it heavily. But there is a mathematical bound to what the discount factor can do! What the assumption would mean is that a damage becomes a gain. Thus, conceptually speaking, people dying in the future coul be made a benfit for today, if only you chose a discount rate high enough. This is nonsense: the discount rates cannot influence real-world outcomes, and impact is an impact: if it reduces welfare in the future, it cannot enhance welfare today by mere discounting. If it could, we could seek to damage future generations as much as we want and always benefit from it by discounting them heavily enough (bear in mind that this benefit were out of the discounted damages, not because of an increase in present consumption!). It doesn’t matter if this damage is net, as the benefit is discounted in the same manner. You can discount the corresponding sum of statistical values of life so much as to make the present value of people dying in the future basically zero. You can’t make people dying in the future a present gain (if it’s no gain in the future; I know of no such assumption). Once you have identified a net damage in the future, the present value by discounting is bounded from below by zero.
c) What I kinda think is what you’re saying now (actually that’s how I interpret it; what you are saying is: LOOK AT THE GRAPH!): by some intricate connections between imputs of an IAM, a higher discount rate makes SCC negative: just LOOK at the graph and let your phantasy loose! And even though you have no clue how this actually happens, and even though you have no prove that it does eventually turn the sign of the average SCC, and even though a direct effect can be excluded, you simply assert that this may be the way how SCC become negative by discounting. This is entirely speculative. There may be some indirect effect of the discount rate. However, this is completely out of line with your original statement and, again, purely speculative. Actually, the fact that the IAMs are blackbox models for us, helps you here: you can just say anything you want. But I can do the same! Now I tell you the following: having an decrease of 19 dollar/tCO2e by going from 2.5 to 3 percent (i.e. a difference quotient of -38), but only a decrease of 22 dollar/tCO2e by going from 3 to 5 percent (i.e. a difference quotient of -11), I “think” (= wildly guess for some reason that has absolutely nothing to do with any evidence provided) that at a certain discount rate, say 7 percent, the change in average SCC as a function of the discount rate turn around and actually INcrease with a higher discount rate. This is based on the exactly same information that you have.
Of course, I do not really believe the latter (even if I worked it out less sloppily): increasing the present value of a future cost by discounting it more is an exceedingly silly idea. But making the discount rate the difference between damage and benefit, is too! What I actually think is happening, is based on an intuitive reading that makes sense, when discounting an actual damage: that the average (etc) damage estimates can decrease to a point that represents the idea that we simply do not care, at all, for a future damage, i.e. that it becomes essentially zero by discounting it heavily enough. For an eventual increase in runs that give negative SCC with higher discount rates, my idea is simply that, as the damage function flattens out, more runs will result in an actual gain, which – in combination with a fat right tail – finally averages the SCC out at zero. Note how this goes nicely with the graph: the higher the discount rate the more narrow the distribution of individual SCC values, with a concentration at lower levels. This is no surprise at all: fully discounted future costs should be zero with high certainty. This poses a problem to your line of reasoning: for some reason, while we are not at all sure about the exact value of the present value of a future damage (huge deviations from the average) when this value is high, we’d be really sure about the present benefit, if somehow the discount rate renders the impacts of AGW so. This makes no sense. Except if you assume that the distribution somehow inverses at zero and flattens again for negative averages, which is a further Deus ex machina kind of assumption combined with the idea that government plus literature are hiding away something that would be obvious, if only they’d run it with a 2 percent points higher discount rate: for no reason a parameter of the distribution would reverse, while the discount rate would increase monotonously.
Shorter abc: If you actually think you have a valid claim that is at odds with a direct assessmant AND that has completely escaped exactly 100 percent off the economists working with those models (plus the government is lying), you should have more evidence than some crude idea based on what is not more than one observation that, that is not based on an understanding of the models, that can not exclude an idea with the opposite conclusion, and that does not explain how the distribution behaves (if it’s not at odds with it). At your (and my) level of understanding, you can report the literature, you cannot criticize it. Therefore, you really really should not teach it. There is no reasoning behind c), it’s just that you boldly trespassed a fool-proof demarcation line of scientific reasonsing by making – as far as it concerns us – an untestable claim. As it stands, this is your last straw following a line of argumentation that was flawed from the very beginning.
OK, I finally understand now what is driving your hostility, Martin. Up till now, I couldn’t even understand what you were thinking of, to be denying the obvious fact that in the diagram, a higher discount rate ==> more runs having negative SCC.
I am going to be doing a full-blown study on this, and I’m getting some of the actual run data from an EPA economist etc., but just to give you a hint:
You’re right that the SCC isn’t directly about the projected path of benefits or harms, given existing levels of emissions. The SCC is a marginal concept.
However, by emitting an additional ton of CO2 today, we *advance* the trajectory of warming; we pull it forward.
So, if a high discount rate (presumably applied to model runs where there aren’t catastrophic impacts in 2085 etc.) means that PDV of total impacts is beneficial, then the SCC would be negative because it brings that stream of net benefits closer to the present, giving a higher PDV.
This is speculative: you have no idea why those model runs got negative, you just assume that it coincides with your made-up idea. I am not sure if the *advancement* of the warming trajectory is actually significant in quantitative terms – did you check that, or is this another ‘it is true because it makes my idea work’ assumption? But let’s assume it is true, the net outcome is ambiguous: it also advances damages, and as those damages are convex (and increasing), the result is therefore not clear, at all. Again, the exact opposite conclusion can be argued for.
In reality, you have not the slightest idea if there is anything about your claim. My hostility comes from the fact, that there is absolutely no demonstration that what you say actually holds under the assumption you advance, even though it goes against everything that has been published on this topic. This topic is extremely polarized, partly because people with little knowledge about the actual literature advance nonsense based on imagination-gone-wild assessments. Yours is a novel one, but given that you have no expertise, you should not make claims based on personal impressions.
Martin wrote:
My hostility comes from the fact, that there is absolutely no demonstration that what you say actually holds under the assumption you advance, even though it goes against everything that has been published on this topic.
Martin, this will be my last volley for now. I just want to point out that you keep alternating between the following evaluations of my position:
(A) It is speculative, and we can’t evaluate it one way or the other, because these models are black boxes to you and me.
(B) It goes against everything in the published literature.
Those are contradictory responses.
b) The idea that a future damage can be turned into a present gain by discounting it heavily. But there is a mathematical bound to what the discount factor can do! What the assumption would mean is that a damage becomes a gain.
No, it’s a matter of taking into account the decades of gains that will precede the future damage. A higher discount rate favors the benefit side of the equation in this case.
If it’s truly just an accident that median and average move significantly to the left and more runs came out negative with increasing discount rate, then the model is not very repeatable, is it.
Right. Just to make sure you get that, Martin, I of course agree with you that a future damage can’t be turned into a positive by bumping up the discount rate. However, if you have a stream of net benefits followed by a stream of net costs, then bumping up the discount rate can obviously render the PDV positive (meaning SCC is negative), even if originally it was negative (meaning SCC is positive), at the lower discount rate. I don’t see why you are disputing this, especially in light of the chart, but I’ve emailed Richard Tol to see if we can get a better answer.
Because your claim is twofold:
a) Refers to your speculation as to why individual runs give negative SCC.
b) Refers to average SCC estimates that you claim can be made negative by chosing a higher discount rate.
a) is speculation, b) is too, b) does not follow from a), your insinutations can serve to come to whatever conlusion one prefers, and you have demonstrated exactly nothing. But you are “teaching” it.
I think that half a degree per century is a significant climate change. It might not be for us, but for the future inhabitants. There are many people that supply their homes with green energy. It might be a big investition, but it worths. “especially if we think we’ll get out of the second Great Depression by 2050” – if you look at the reality into its face you will see that things are going to this directoin. Detroit announced a bankruptcy! But we still are waiting to get out of the crises doing nothing.
I agree with Martin: there is *absolutely* no way that discounting harm in the future could ever turn into a benefit today. Agreed. You are 100% right on that, Martin.
Bob’s point, though, is that we could avoid that harm in the future by paying a price now. In order to determine whether that would be a gainful or lossy transaction, we must determine the current price of the future harm. If the harm is (as the Native Americans say) eight generations in the future, the current price is zero. That is, paying *any* price results in a complete loss.
Will the harm happen in seven generations? Well, then it’s worth spending a little bit to avoid it, but not much. If we propose to spend a huge amount now to avoid a small harm then, it’s *quite* possible for the transaction to come up negative.
Does this explanation help, Martin? I know that it’s a difficult concept — that we should discount future events — but stick with it, and eventually you’ll learn to think like an economist! Good luck!